



Previously published in the Bulletin of the Scientific Instrument Society No.115, December 2012 and the Slide Rule Gazette Issue 14, Autumn 2013
The story starts with my inheriting an alcohol slide rule from my late father in law some 21 years ago. He was an architectural draughtsman and surveyor and had worked for Kemp Town Brewery in Brighton, later Charrington’s Brewery in Newhaven. When the Kemp Town Brewery closed he saved a number of items that would otherwise have been thrown away including this slide rule.
In 1995 I started to collect drawing instruments and slide rules and at a subsequent visit to the Science Museum in London, soon after, saw a different slide rule by the same maker (Cock) in their collection and was surprised to find that it dated from the second quarter of the nineteenth century. At this stage I had no idea, however, that it was a rather unusual one.
Like the rest of my collection, I photographed it and, a few years ago, put a picture of it on my website. A year or two ago, Thomas Wyman, an American slide rule collector and former president, now president emeritus of the Oughtred Society, contacted me to ask if he could use images of the slide rule for an article he was writing. I also took the slide rule to the Members’ Day in May and Louise Muse spotted it, commented that she had not seen another like it, and suggested I write about it for the Bulletin. Well here it is.
Before considering the missing link we need to look briefly at the origins and development of the gauger’s slide rule up to the introduction of Imperial measures. The invention of the gauger’s slide rule is generally credited to Thomas Everard in 1683 and described in his work on Gauging. Originally this rule had two slides on opposite faces. Over the years it was developed until it had three slides, leaving just one face plain and then four slides, one on each face of a rule that was nearly square in cross-section. This type was the standard gauger’s slide rule for many years.
The three slide version was described by another gauger, Charles Leadbetter. The four slide version appeared around the middle of the eighteenth century and it is quite common today. In 1824 the Imperial gallon was introduced and replaced the old wine and ale gallons as the standard in Britain from that date. The standard form of gauger’s slide rule continued to be the approximately square section one with a slide on each face, only the gauge points being changed.
This type of slide rule was made or sold by, amongst other makers, S Cock of Store Office, Excise Office, Broad Street, London. Clifton gives a single date of 1840 for this address but judging by the number and variety of slide rules and other items found with his name on he must have been active for rather more than one year.
This rule, from my collection, is illustrated in Figures 1 to 4 and is absolutely typical of the post 1824 four slide type with the Imperial gauge marks. It is twelve inches long.
In 1995 I started to collect drawing instruments and slide rules and at a subsequent visit to the Science Museum in London, soon after, saw a different slide rule by the same maker (Cock) in their collection and was surprised to find that it dated from the second quarter of the nineteenth century. At this stage I had no idea, however, that it was a rather unusual one.
Like the rest of my collection, I photographed it and, a few years ago, put a picture of it on my website. A year or two ago, Thomas Wyman, an American slide rule collector and former president, now president emeritus of the Oughtred Society, contacted me to ask if he could use images of the slide rule for an article he was writing. I also took the slide rule to the Members’ Day in May and Louise Muse spotted it, commented that she had not seen another like it, and suggested I write about it for the Bulletin. Well here it is.
Before considering the missing link we need to look briefly at the origins and development of the gauger’s slide rule up to the introduction of Imperial measures. The invention of the gauger’s slide rule is generally credited to Thomas Everard in 1683 and described in his work on Gauging. Originally this rule had two slides on opposite faces. Over the years it was developed until it had three slides, leaving just one face plain and then four slides, one on each face of a rule that was nearly square in cross-
The three slide version was described by another gauger, Charles Leadbetter. The four slide version appeared around the middle of the eighteenth century and it is quite common today. In 1824 the Imperial gallon was introduced and replaced the old wine and ale gallons as the standard in Britain from that date. The standard form of gauger’s slide rule continued to be the approximately square section one with a slide on each face, only the gauge points being changed.
This type of slide rule was made or sold by, amongst other makers, S Cock of Store Office, Excise Office, Broad Street, London. Clifton gives a single date of 1840 for this address but judging by the number and variety of slide rules and other items found with his name on he must have been active for rather more than one year.
This rule, from my collection, is illustrated in Figures 1 to 4 and is absolutely typical of the post 1824 four slide type with the Imperial gauge marks. It is twelve inches long.

Fig 1 - Cock rule of the Everard type, face 1

Fig 2 - Face 2

Fig 3 - Face 3

Fig 4 - Face 4
Let’s look at the scales on each face in turn. Face 1, which bears the gauge points IMB and IMG has a scale labelled ‘A’ on the upper stock that is a log scale of single radius from 1 to 10. The slide has a scale labelled ‘B’ that is also a single radius log scale but it starts some distance in and so runs from 1 to 9.5. In other respects it corresponds to the ‘A’ scale so the two together could be used for multiplication. On the lower stock of this first face is a reciprocal, single radius, log scale with a displaced start running (right to left) from 2.5 to 25.
On each of the first three rules to be discussed there are two slide faces with the late starting log scale but not always in relation to the same stock scales; it is likely that the slides have been removed at some time from these rules and reinserted differently. In fact this is very likely as all the first three rules exhibit an improvement by a gauger by the name of Vero; inserting a pair of single radius slides in any groove (with the one ending in 9.5 on the left and the other type on the right) creates a long double radius slide, avoiding the need to move the slide end to end in some calculations.
Face 2, for segment lying calculations, has a partial log scale on the upper stock running from 1 to 4. The slide has a scale labelled ‘B’ that is a single radius log scale from 1 to 10. The lower stock has another partial log scale running from 4 to 10.
Face 3 bears the gauge point IMG. On the upper stock is a half radius log scale running from 1 to 3.2. The slide has a scale labelled ‘B’ and log 1 to 9.5 (see above). On the lower stock is a half radius log scale from 3.2 to 10. In fact setting the slide so that the one coincides with the one on the upper scale the ten on the slide corresponds with 3.16 on the upper scale which is √10 so the relationship between the scales on the stock and slide gives squares/square roots. This is the same on the next two rules.
Face 4, for segment standing calculations, has a partial log scale running from 1 to 8 on the upper stock. The ‘C’ scale on the slide is single radius log from 1 to 10. The lower stock scale is a log one from 8 to 100.
On the underside of the slide in face one are scales for correcting the calculations for casks of the spheroid and 2nd varieties.
Now we must turn our attention to the rule that came from the Kemp Town Brewery. This is another rule by the same maker, S Cock but now the four slides are located on the top and bottom faces, two on each face and the rule is much more rectangular in section. It is nine inches long and has brass strengthening plates on each end. This is illustrated in Figures 5 to 9.
On each of the first three rules to be discussed there are two slide faces with the late starting log scale but not always in relation to the same stock scales; it is likely that the slides have been removed at some time from these rules and reinserted differently. In fact this is very likely as all the first three rules exhibit an improvement by a gauger by the name of Vero; inserting a pair of single radius slides in any groove (with the one ending in 9.5 on the left and the other type on the right) creates a long double radius slide, avoiding the need to move the slide end to end in some calculations.
Face 2, for segment lying calculations, has a partial log scale on the upper stock running from 1 to 4. The slide has a scale labelled ‘B’ that is a single radius log scale from 1 to 10. The lower stock has another partial log scale running from 4 to 10.
Face 3 bears the gauge point IMG. On the upper stock is a half radius log scale running from 1 to 3.2. The slide has a scale labelled ‘B’ and log 1 to 9.5 (see above). On the lower stock is a half radius log scale from 3.2 to 10. In fact setting the slide so that the one coincides with the one on the upper scale the ten on the slide corresponds with 3.16 on the upper scale which is √10 so the relationship between the scales on the stock and slide gives squares/square roots. This is the same on the next two rules.
Face 4, for segment standing calculations, has a partial log scale running from 1 to 8 on the upper stock. The ‘C’ scale on the slide is single radius log from 1 to 10. The lower stock scale is a log one from 8 to 100.
On the underside of the slide in face one are scales for correcting the calculations for casks of the spheroid and 2nd varieties.
Now we must turn our attention to the rule that came from the Kemp Town Brewery. This is another rule by the same maker, S Cock but now the four slides are located on the top and bottom faces, two on each face and the rule is much more rectangular in section. It is nine inches long and has brass strengthening plates on each end. This is illustrated in Figures 5 to 9.
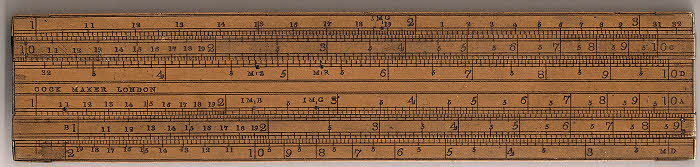
Fig 5 - Cock slide rule with two slides on the upper face and two on the lower face; face 1
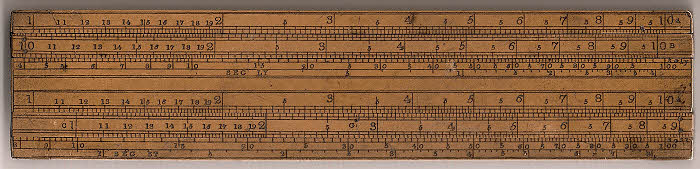
Fig 6 - Face 2

Fig 7 - Edge 1
Fig 8 - Edge 2
Fig 9 - Slide under side
Let’s now look at the scales on this slide rule. Face 1 can be considered as two separate parts, upper and lower. The upper part has the same scales and gauge point on the stock as the third face of the ‘Everard’ type rule. The slide has a single radius log scale from 1 to 10. The lower part has the same gauge points and scales on the stock as the first face on the ‘Everard’ type rule and the same 1 to 9.5 single radius log scale on the slide.
Face 2 can again be considered as two parts. The upper part (segment lying) has an upper stock scale labelled ‘A’ that is a single radius log scale from 1 to 10. The slide has a corresponding ‘B’ scale also single radius 1 to 10. The lower part of the stock (upper part) has two log scales running from 4 to 100 and 1 to 4. These are a modified form of the scales on face 2 of the ‘Everard’ type rule. The lower part is for segment standing and is similar to the upper part except that the scales on the lower part of the stock run from 1 to 8 and 8 to 100 and the slide runs from 1 to 9.5. These are a modified form of the scales on face 4 of the ‘Everard’ type rule. As noted above the slides in the upper part of face 1 and the lower part of face 2 have probably been swapped over at some time. This only became apparent when I started comparing the rules in detail.
The first edge shown has a series of gauge points listed in a table. The other edge has two log scales one of which is a single radius log scale from 1 to 10, the other being another single radius log scale of the same physical length but displaced to read from 1.6 to 16.
Two slides are plain on their undersides, one has a scale of 0 to 9 inches and two scales for cask shapes (spheroid and 2nd variety), and the last has a scale from 9 to 18 inches.
The third rule is unsigned but a similar rule, signed Cock, exists in Thomas Wyman’s collection. This is an open frame boxwood rule, again 9 inches long, with brass bands holding the three parts of the stock together. It is illustrated in Figures 10 and 11.
Face 2 can again be considered as two parts. The upper part (segment lying) has an upper stock scale labelled ‘A’ that is a single radius log scale from 1 to 10. The slide has a corresponding ‘B’ scale also single radius 1 to 10. The lower part of the stock (upper part) has two log scales running from 4 to 100 and 1 to 4. These are a modified form of the scales on face 2 of the ‘Everard’ type rule. The lower part is for segment standing and is similar to the upper part except that the scales on the lower part of the stock run from 1 to 8 and 8 to 100 and the slide runs from 1 to 9.5. These are a modified form of the scales on face 4 of the ‘Everard’ type rule. As noted above the slides in the upper part of face 1 and the lower part of face 2 have probably been swapped over at some time. This only became apparent when I started comparing the rules in detail.
The first edge shown has a series of gauge points listed in a table. The other edge has two log scales one of which is a single radius log scale from 1 to 10, the other being another single radius log scale of the same physical length but displaced to read from 1.6 to 16.
Two slides are plain on their undersides, one has a scale of 0 to 9 inches and two scales for cask shapes (spheroid and 2nd variety), and the last has a scale from 9 to 18 inches.
The third rule is unsigned but a similar rule, signed Cock, exists in Thomas Wyman’s collection. This is an open frame boxwood rule, again 9 inches long, with brass bands holding the three parts of the stock together. It is illustrated in Figures 10 and 11.
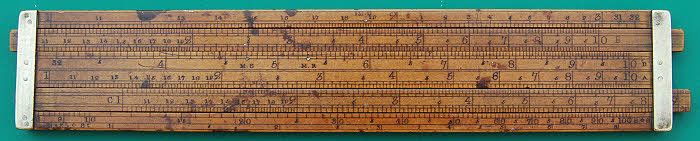
Fig 10 - Open frame gauger’s slide rule face 1
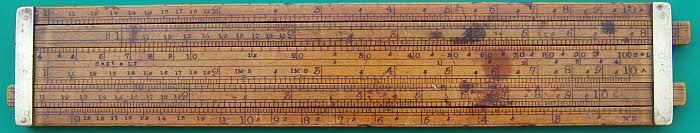
Fig 11 - Open frame gauger’s slide rule face 2
The order of the four parts is different again but, as we shall see, they are the same as on the previous rule by Cock although once again the slides have been removed and differently replaced at some time. Comparison with a similar rule signed Grove illustrated in a paper by Peter Hopp suggests that the slides have been interchanged and that the position of the slides with scales ending at 9.5 should be as on the ‘Everard’ type slide rule, if indeed there actually is a ‘correct’ position.
The first face upper part has first a half radius log scale from 1 to 3.2 and then a single radius log scale from 1 to 10 on the slide, whilst below the slide is a half radius log scale from 3.2 to 10 as on the upper part of the first face of the second rule. The lower part has the segment standing scales that are the same as on the lower part of the second face of the second rule.
On the second face the upper part has the segment lying scales that are the same as on the upper part of second face of the second rule, whilst the lower part has the same scales on the stock of the lower part of face one of the second rule with a single radius log scale on the slide from 1 to 9.5.
There are two sets of edge scales. On one edge there is a single radius log scale from 1 to 10 with an adjacent log scale running from 1.2 to 12. On the other there are again two adjacent single radius log scales but this time running from 1 to 10 and 1.6 to 16. With these scales, reading from one to the adjacent one enables multiplication by factors of 12 and 16 respectively and these are common factors in imperial coinage and weights & measures. (e.g. shillings to pence and pounds to ounces).
So far, taking the four sets of scales on each of these rules, they are the same except for the order in which they are placed once the slides are re-located in the second and third rules. The fourth rule I am about to discuss is however rather different. The reason for including it will, I hope, become clearer later.
The first face upper part has first a half radius log scale from 1 to 3.2 and then a single radius log scale from 1 to 10 on the slide, whilst below the slide is a half radius log scale from 3.2 to 10 as on the upper part of the first face of the second rule. The lower part has the segment standing scales that are the same as on the lower part of the second face of the second rule.
On the second face the upper part has the segment lying scales that are the same as on the upper part of second face of the second rule, whilst the lower part has the same scales on the stock of the lower part of face one of the second rule with a single radius log scale on the slide from 1 to 9.5.
There are two sets of edge scales. On one edge there is a single radius log scale from 1 to 10 with an adjacent log scale running from 1.2 to 12. On the other there are again two adjacent single radius log scales but this time running from 1 to 10 and 1.6 to 16. With these scales, reading from one to the adjacent one enables multiplication by factors of 12 and 16 respectively and these are common factors in imperial coinage and weights & measures. (e.g. shillings to pence and pounds to ounces).
So far, taking the four sets of scales on each of these rules, they are the same except for the order in which they are placed once the slides are re-
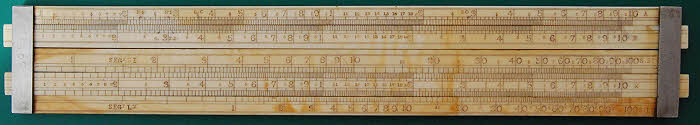
Fig 12 - Ivory gauging slide rule signed J Long Maker 43 East Cheap London, face 1

Fig 13 - Ivory gauging slide rule signed J Long Maker 43 East Cheap London, face 2
This is the newest of the four slide rules discussed in this paper. The firm of Joseph Long (posthumously) was at this address from 1885 to 1936. Whilst primarily a gauging rule it is also a comparative rule.
Consider first the upper part of face 1. There are just two scales, one on the stock above the slide and one on the slide. Both are double radius log scales from 1 to 100. The lower part allows calculations for both segment lying and segment standing, thus combining the functions of two faces on the ‘Everard’ type rule into one. All three scales, on the slide and on the stock both sides of the slide are double radius log scales from 1 to 100 but the scales either side of the slide are shorter and both of different lengths. This is effectively an inversion of the arrangement on the other rules where the stock scales were longer and too long to fit on the rule in one pass.. On this fourth rule the slide functions as the stock on the others and vice versa.
On the second face the upper part has just two scales, one on the stock labelled ‘D’ is a single radius log scale from 1 to 10, whilst the ‘C’ scale on the slide is a double radius one from 1 to 100. This would perform the same function as the part on the other rules with the stock scale divided above and below the slide at 3.2. The lower part is an addition to the functions on the ‘Everard’ type rule with a ‘comparative’ function. It’s scales are ‘E’, a 200 to 20 reciprocal log scale above the slide, an 80 -0 -70 proof scale on the slide, and ‘F’ a log scale from 3 to 30.
Why have I included this slide rule? Firstly it is a further progression from rule three. However, in his paper that I have already mentioned, Thomas Wyman postulates that the transition from the ‘Everard’ type of excise slide rule of almost square cross-section to the open frame type resulted from the example of the Soho slide rule and he describes other transitional excise slide rules to further illustrate this. However, in my opinion, the transition to the use of double radius log scales exampled by this last slide rule is an equally significant change, quite possibly influenced by Watt’s Soho slide rule that had three double radius log scales and one single radius one, although Leadbetter’s rule with three slides also had double radius scales as did the original two slide Everard gauging slide rule according to Stone’s translation of Bion so it may just have been a revival from these earlier gauging slide rules, which pre-date the Soho slide rule, and a rejection of Vero’s improvement.
In conclusion I believe that the second slide rule in this paper is a rare example of type of slide rule that was produced for a short period of time, probably about 1840 and is a link between the ‘Everard’ type of slide rule and the open frame type. The fact that all three types signed by Cock are known reinforces this conclusion. Hence my christening it the ‘Missing Link’.
Notes and References
Consider first the upper part of face 1. There are just two scales, one on the stock above the slide and one on the slide. Both are double radius log scales from 1 to 100. The lower part allows calculations for both segment lying and segment standing, thus combining the functions of two faces on the ‘Everard’ type rule into one. All three scales, on the slide and on the stock both sides of the slide are double radius log scales from 1 to 100 but the scales either side of the slide are shorter and both of different lengths. This is effectively an inversion of the arrangement on the other rules where the stock scales were longer and too long to fit on the rule in one pass.. On this fourth rule the slide functions as the stock on the others and vice versa.
On the second face the upper part has just two scales, one on the stock labelled ‘D’ is a single radius log scale from 1 to 10, whilst the ‘C’ scale on the slide is a double radius one from 1 to 100. This would perform the same function as the part on the other rules with the stock scale divided above and below the slide at 3.2. The lower part is an addition to the functions on the ‘Everard’ type rule with a ‘comparative’ function. It’s scales are ‘E’, a 200 to 20 reciprocal log scale above the slide, an 80 -
Why have I included this slide rule? Firstly it is a further progression from rule three. However, in his paper that I have already mentioned, Thomas Wyman postulates that the transition from the ‘Everard’ type of excise slide rule of almost square cross-
In conclusion I believe that the second slide rule in this paper is a rare example of type of slide rule that was produced for a short period of time, probably about 1840 and is a link between the ‘Everard’ type of slide rule and the open frame type. The fact that all three types signed by Cock are known reinforces this conclusion. Hence my christening it the ‘Missing Link’.
Notes and References
1. Thomas Wyman, SOHO Steam Engines, The First Engineering Slide Rule and the Evolution of Excise Rules, Proceedings of the 18th International Meeting of Slide Rule Collectors, pp 63-72
2. Florian Cajori, A History of the Logarithmic Slide Rule, 1910, Astragal Press reprint 1994, p17
3. Thomas Everard, Stereometry made easie, London, 1684
4. Charles Leadbetter, The Royal Gauger: or Gauging made Easy, 1739
5. Gloria Clifton, Directory of British Scientific Instrument Makers 1550-1851, Zwemmer, 1995
6. Peter M Hopp, Alcohol Slide Rules, Slide Rule ’99 display article available on http://sliderules.lovett.com
7. Thomas Wyman, op cit, p71 “While the timing may seem a coincidence, it does appear that in commissioning the design and production of the engineering slide rule sometime about 1778, Watt unintentionally triggered fundamental design changes in the special-purpose slide rules used by Officers of the Excise.”
8. Charles Leadbetter, The Royal Gauger,p27 for example “On the first face of the Instrument are placed four lines of numbers, the first line of numbers consists of two radiuses, and is numbered 1, 2, 3, 4, 5, 6, 7, 8, 9, 10 and then 2, 3, 4, 5, 6, 7, 8,9, 10.”
2. Florian Cajori, A History of the Logarithmic Slide Rule, 1910, Astragal Press reprint 1994, p17
3. Thomas Everard, Stereometry made easie, London, 1684
4. Charles Leadbetter, The Royal Gauger: or Gauging made Easy, 1739
5. Gloria Clifton, Directory of British Scientific Instrument Makers 1550-
6. Peter M Hopp, Alcohol Slide Rules, Slide Rule ’99 display article available on http://sliderules.lovett.com
7. Thomas Wyman, op cit, p71 “While the timing may seem a coincidence, it does appear that in commissioning the design and production of the engineering slide rule sometime about 1778, Watt unintentionally triggered fundamental design changes in the special-
8. Charles Leadbetter, The Royal Gauger,p27 for example “On the first face of the Instrument are placed four lines of numbers, the first line of numbers consists of two radiuses, and is numbered 1, 2, 3, 4, 5, 6, 7, 8, 9, 10 and then 2, 3, 4, 5, 6, 7, 8,9, 10.”
9. Edmund Stone’s translation of M. Bion, The Construction and Principal Uses of Mathematical Instruments, London, 1758, reprinted by the Astragal Press in 1995
| Early Sets |
| Traditional Sets |
| Later Sets |
| Major Makers |
| Instruments |
| Miscellanea |
| W F Stanley |
| A G Thornton |
| W H Harling |
| Elliott Bros |
| J Halden |
| Riefler |
| E O Richter |
| Kern, Aarau |
| Keuffel & Esser |
| Compasses |
| Pocket compasses |
| Beam compasses |
| Dividers |
| Proportional dividers |
| Pens |
| Pencils |
| Rules |
| Protractors |
| Squares |
| Parallels |
| Pantographs |
| Sectors |
| Planimeters |
| Map Measurers |
| Miscellaneous |
| Materials Used |
| Who made them |
| Who made these |
| Addiator |
| Addimult |
| Other German |
| USA |
| Miscellaneous |
| Microscopes |
| Barometers |
| Hydrometers & Scales |
| Pedometers |
| Other instruments |
| Workshop Measuring Tools |
| Catalogues & Brochures |